
The Rubik's Cube is a famous puzzle game that was invented in 1974 by Erno Rubik from Hungary. Since then, the game became popular and became known in every corner of the world.
Because it is a game of some difficulty and requires great skill and mental ability, everyone started playing it, and the cube began to be the subject of scientific analysis.
In 1981, the mathematician Morwen Thistlethwaite, from the University of Tennessee in the United States, managed to show the world that this game could always be solved in less than 52 moves, no matter what order the cube was in.
In 1992, the Dutchman Hans Kloosterman managed to reduce this limit, showing that any situation in the Rubik's Cube could be solved in less than 42 moves. But in 2008, the Americans again reduced this record, and managed to show that the Rubik's Cube could be solved in 22 moves. In 2010, a group of scientists from around the world proved that the number was even lower: the puzzle can always be solved in 20 moves or less.
The cube can have 43,252,003,274,489,856,000 different orderings, different combinations. This shows how difficult it is to perform these calculations and arrive at exact figures. However, modern mathematics and technology have allowed us to reach this point of analysis. Are you still waiting for someone to tell you how to solve the Rubik's cube?
Number of possible combinations.

We can combine all the vertices of the cube with each other in any way, which gives rise to8!,!possibilities. The same happens with the edges; that is, we can combine them as we wish, which gives rise to12!,!possibilities, but the total permutation of vertices and edges must be even, which eliminates half of the possibilities. On the other hand, we can rotate all the vertices as we wish except one without changing anything else in the cube. The orientation of the last vertex will be determined by the orientation of the other seven, and this creates3^7,!possibilities for us. The same must happen with the edges, since2^{11},!more possibilities appear. In total we will have that the number of possible permutations in the Rubik's Cube is:
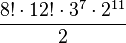
That is, forty-three trillion two hundred fifty-two thousand three billion two hundred seventy-four thousand four hundred eighty-nine million eight hundred fifty-six thousand permutations.
There are many variations of the original Rubik's Cube and also similar puzzles, cubic or otherwise. Among the cubic variations, the "Magic Cube" stands out, which is mechanically identical to the original, but uses colored numbers on its faces in such a way that the only way to solve it is if all the numbers are right-side up on the same face, additionally the numbers on the faces form magic squares which can all have the same constant. A very similar cube is the Cuboku, in which the objective is to form sudokus with the numbers on the faces. Or a cube cut in a way that is not parallel to the faces: the Skewb.
Others include placing pictures instead of colors or color designs that confuse the solver, such as placing four different colors on each face of a 4x4x4 for a total of 24 different colors. Or reducing the number of colors to 3.
Non-cubic shapes include extended cubes that have one or more additional layers, which may be fully or partially functional. There are also puzzles based on world maps and other Platonic solids: the Skewb Diamond, the Megaminx, the Pyraminx or the Dogic among others. For most of these variations it is possible to think of other puzzles that are broken into a larger number of pieces in the same way as Rubik's Revenge for example, as well as different ways to color them.
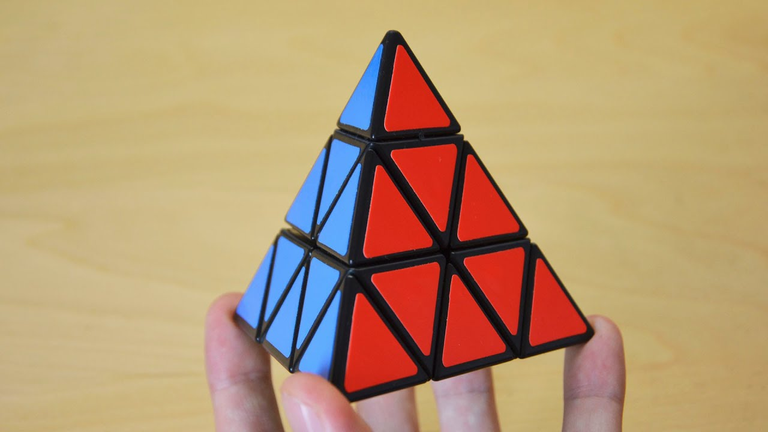
Pyraminx solved .

During the cube's heyday, video game company Atari released its cartridges for the Atari 2600 console called "Rubik's Cube" (CX2698), "Atari Video Cube" (a reissue that changed its name for copyright reasons, CX2670) and the prototype "Rubik's Cube 3D" that was never released.
Extradimensional variations.

In 1994 Melinda Green, Don Hatch, and Jay Berkenilt created the so-called "MagicCube4D", which is a 4-dimensional analogue of the Rubik's Cube in Java consisting of hypercubes from 2x2x2x2 to 5x5x5x5. With many more possible states this object is much more difficult to solve. So far only 78 people have managed to solve it. The geometric shape of this cube is a tesseract, which has each line divided into 3 equal parts for the standard puzzle, the result of this is that in addition to the 1, 2, and 3 colored pieces of the 3-dimensional cube there is a fourth type of piece with 4 colors each, which are at the vertices.
In 2006 Roice Nelson and Charlie Nevill created the 5-dimensional "Magic Cube 5D" model from 2x2x2x2x2 to 5x5x5x5x5 which so far has been solved by only twelve people. In this puzzle there are also pieces with five colors which are also on the vertices.

El cubo de Rubik es un famoso juego tipo Puzzle que fue inventado en el año 1974 por Erno Rubik, de Hungría. Desde ese momento el juego se popularizó y llegó a ser conocido en todos los rincones del mundo.
Debido a que es un juego de cierta dificultad y que requiere de gran destreza y capacidad mental, todos empezaron a jugarlo, y el cubo empezó a ser objeto de análisis de los científicos.
En 1981, el matemático Morwen Thistlethwaite, de la universidad de Tenesse en los Estados Unidos, logró demostrar al mundo que este juego podía ser siempre resuelto en menos de 52 movimientos, no importaba cual fuera el orden en el que se encontrara el cubo.
En 1992 el holandés Hans Kloosterman logró reducir este límite, demostrando que cualquier situación en el cubo de Rubik podría ser resuelta en menos de 42 movimientos. Pero en 2008 de nuevo los estadounidenses redujeron este récord, y lograron mostrar que el Cubo de Rubik podría ser resuelto en 22 jugadas. En el año 2010, un grupo de científicos de todo el mundo, demostraron que eran aún menos: Siempre se puede resolver el puzzle con 20 jugadas o menos.
El cubo puede tener 43.252.003.274.489.856.000 formas de orden diferentes, combinaciones distintas. Esto demuestra la dificultad para realizar estos cálculos y llegar a cifras exactas. Sin embargo, las matemáticas modernas y la tecnología han permitido llegar a este punto de análisis. Y tú, ¿todavía esperas que alguien te diga cómo resolver el cubo de Rubik?
Número de combinaciones posibles.

Podemos combinar entre sí de cualquier forma todos los vértices del cubo, lo que da lugar a 8!,! posibilidades. Con las aristas pasa lo mismo; es decir, que podemos combinarlas como se desee, lo que da lugar a 12!,! posibilidades, pero la permutación total de vértices y aristas debe de ser en total par, lo que nos elimina la mitad de las posibilidades. Por otra parte, podemos rotar todos los vértices como queramos salvo uno sin cambiar nada más en el cubo. La orientación del último vértice vendrá determinada por la que tengan los otros siete, y esto nos crea 3^7,! posibilidades. Igual debe ocurrir con las aristas, pues aparecen 2^{11},! posibilidades más. En total tendremos que el número de permutaciones posibles en el Cubo de Rubik es de:
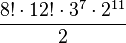
Es decir, cuarenta y tres trillones doscientos cincuenta y dos mil tres billones doscientos setenta y cuatro mil cuatrocientos ochenta y nueve millones ochocientas cincuenta y seis mil permutaciones.
Existen muchas variaciones del Cubo de Rubik original y también de rompecabezas parecidos, de forma cúbica o de otras distintas. Entre las variaciones cúbicas destaca el "Cubo Mágico" el cual es mecánicamente idéntico al original, pero usa números de colores en sus caras de tal manera que la única forma de resolverlo es que todos los números estén al derecho en la misma cara, adicionalmente los números de las caras forman cuadrados mágicos los cuales pueden tener todos la misma constante. Un cubo muy similar es el cuboku en el cual el objetivo es formar sudokus con los números de las caras. O un cubo cortado de manera no paralela a las caras: el Skewb.
Otras incluyen colocar imágenes en lugar de colores o diseños de colores que confundan al que resuelve, como colocar en un 4x4x4 cuatro colores distintos en cada cara para un total de 24 colores distintos. O también reducir el número de colores a 3.
Entre las formas no cúbicas destacan los cubos extendidos que tienen una o más capas adicionales, las cuales pueden ser completa o parcialmente funcionales. También están los rompecabezas basados en mapamundis y otros sólidos platónicos: el Skewb diamante, el Megaminx, el Pyraminx o el Dogic entre otros. Para la mayoría de estas variaciones es posible pensar en otros rompecabezas que estén partidos en un mayor número de piezas de la misma manera que La Venganza de Rubik por ejemplo, así como distintas maneras de colorearlos.
Pyraminx resuelto.
Durante el auge del cubo, la empresa de videojuegos Atari lanzó sus cartuchos para consola Atari 2600 llamados "Rubik's Cube" (CX2698), "Atari Video Cube" (reedición que cambió el nombre por razones de copyright, CX2670) y el prototipo "Rubik's Cube 3D" que no salió al mercado.
Variaciones extra dimensionales.

En 1994 Melinda Green, Don Hatch, y Jay Berkenilt crearon el llamado "MagicCube4D", el cual es un modelo tetradimensional análogo de el Cubo de Rubik en Java el cual consiste en hipercubos desde 2x2x2x2 hasta 5x5x5x5. Con muchos más estados posibles este objeto es mucho más difícil de resolver. Hasta ahora sólo 78 personas lo han conseguido resolver. La forma geométrica de este cubo es de un teseracto, el cual tiene cada línea dividida en 3 partes iguales para el rompecabezas estándar, el resultado de esto es que además de las piezas de 1, 2, y 3 colores del cubo de 3 dimensiones existe un cuarto tipo de pieza con 4 colores cada una, las cuales están en los vértices.
En 2006 Roice Nelson y Charlie Nevill crearon el modelo pentadimensional "Magic Cube 5D" desde 2x2x2x2x2 hasta 5x5x5x5x5 que hasta ahora ha sido resuelto sólo por doce personas. En este rompecabezas existen además piezas con cinco colores las cuales están también sobre los vértices.
Source images / Fuente imágenes: Rubik.


Gracias, pero ahora que tenemos la solución, los solucionadores no parecerán tan inteligentes. lol
Nunca supe como lo hace pero mi nieto lo resuelve en un par de minutos. Instinto.
Lástima que más gente no tuviera el instinto de usar Linux
Más que instinto perseverancia. Y, en cierto modo, una manera de limitar el superpoder dse las multinacionales del software. Pero se hace todo a pulmón y es muy difícil perseverar.
Gracias por comentar e interesarte sobre el tema.
De nalgas