"The term 'Delta' is commonly used in trading, especially in the context of stocks or cryptocurrencies derivatives. It is important to understand its meaning and application in real trading, even if you do not participate in derivatives trading."
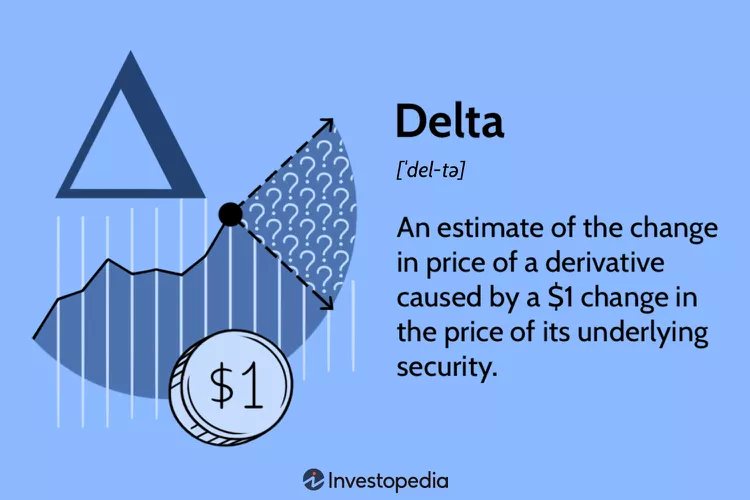
In derivatives trading terminology, "delta" refers to the sensitivity of the derivative price to changes in the price of the underlying asset. For example, an investor purchases 20 call option contracts on a stock. If the stock goes up by 100% but the value of the contracts only increases by 75%, the delta for the options will be 0.75.
Call option deltas are positive, while put option deltas are negative.
Delta measures the change in option premium generated by a change in the underlying security. Delta's value ranges from -100 to 0 for puts and 0 to 100 for calls (multiplied by 100 to move the decimal). Puts generate negative delta because they have a negative relationship to the underlying security i.e. put prices fall when the underlying rises and vice versa.
On the other hand, call options generate a positive relationship with the underlying security's price. So, if the underlying goes higher so does the call premium, as long as other variables that include implied volatility and time remaining until expiration remain constant. Conversely, if the underlying price falls, the call premium will also fall, as long as other variables remain constant.
An at-the-money option typically has a delta of approximately 50, which means that the option premium will rise or fall by approximately one-half point in response to a one-point move up or down in the underlying security. For example, if an at-the-money wheat call option has a delta of 0.5, and the price of wheat increases by 10 cents, the premium will increase by approximately 5 cents (0.5 x 10 = 5), or $250 (since each cent in premium is worth $50).
Source: Investopedia